Find the speed of. If the system is released from rest determine the velocity of block A after it has moved 2 m.
Max either one alone will not slip 2 3 2 2max 12.
. A Determine the possible values for the magnitude of jPjthat allow the block to remain stationary. A horizontal applied force of 12 N and a vertical applied force of 15 N act on the block as shown above. How much work is done by the force as the block moves from the origin to x 80 m.
The block is pulled 40mm down from its equilibrium position and released. A 50kg block moves between vertical guides as shown. The tension in the wire is T see figure.
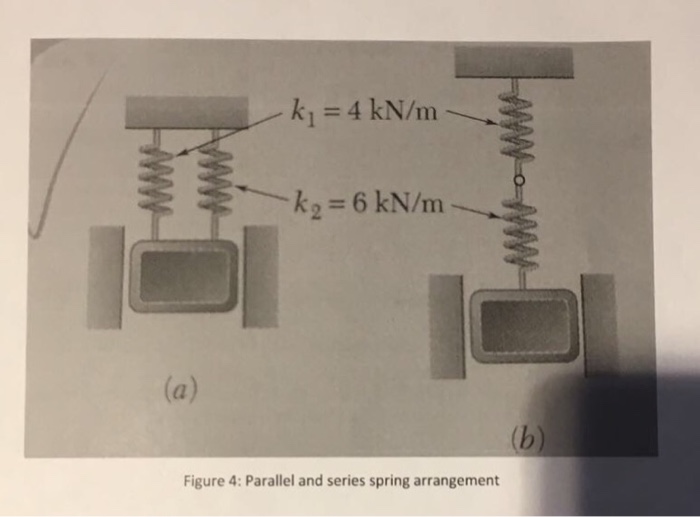
A 10-kg block is pushed against a vertical wall by a horizontal force of 100 N as shown in the figure. A block of unknown mass is attached to a spring with k65 N m. For each spring arrangement determine a the period of the vibration b the maximum velocity of the block and c the maximum acceleration of the block.
Two weights are connected by a massless wire and pulled upward with a constant speed of 150 ms by a vertical pull P. The block is pulled 40mm down from its equilibrium position and released. The block is pulled 40mm down from its equilibrium position and released.
5040 kg-blocks tend to move together F. For each spring arrangement determine a the period of the vibration b the maximum velocity of the block and c the maximum acceleration of the block. The coefficient of static friction µs between the block and the wall is 060.
A 150 kg block is initially at rest. The coefficient of friction between the 6 k g block and the surface is 0. For each spring arrangement.
SOLUTION a Simple harmonic motion. Up to 24 cash back A 50-kg block moves between vertical guides as shown. A 50-kg block is pushed a distance of 50 m across a floor by a horizontal force Fp whose magnitude is 150 N.
Assume that the coefficient of friction between block A and the plane is µk 025 and that the pulley is weightless and frictionless. A 400-kg block rests between the floor and a 300-kg block as shown in the figure. Answer 1 of 9.
Fp is parallel to the displacement of the block. For each spring arrangement determine the period of vibration. A 4 k g block is kept on a top of 6 k g block which is resting on a horizontal surface.
How much work is done by the force as the block moves from the origin to x 8. It undergoes SHM with an amplitude of 10cm. A 50 kg block moves in a straight line on a horizontal frictionless surface under the influence of a force that varies with position as shown.
If there is no maximum. A 50-kg block moves between vertical guides as shown. Between the block and the surface it slides upon is 0350.
Compared to the power developed by car B what is the power. N 1200 N cos 25 750 Nsin 25. Then it is pulled by a rope with constant tension of 200 N as shown.
The spring whose spring constant is 198Ncm is. T p n 2 28 284 0 22214s t n 0 222s f n n 11 0 22214 450 tHz b x xt m 50 0 05 005. For each spring arrangement determine.
Apply the principle of work and energy separately to blocks A and B. The coefficient of kinetic friction is 025. F 1200 N sin 25 750 N cos 25 0 F 1726 N F 1726 N ΣFy 0.
The scale of the figures vertical axis is set by Fs 15 N. F 2 4 6 8 Position m Number i Units. For each spring arrangement determine a the period of the vibration b the maximum velocity of the block and c the maximum acceleration of the block.
P F F 50gsin30 0 P 938 N 222. A 200 kg block is placed against a spring on a frictionless 26 incline. From its equilibrium position and released.
And the coefficient of kinetic friction µk is 040. The block is pulled 40 mm down from its equilibrium position andreleased. For each spring arrangement determine.
A 14-kg block is supported as shown by a spring of constantk 400 Nm which can act in tension or compression. Which one of the following statements is true if the block is initially at rest. Car A moves up the hill at a constant speed that is three times the constant speed of car B.
The block is pulled 40mm down from its equilibrium position and released. Resulting motion b the maximum velocity and acceleration of the block if the amplitude of its motion is 50 mm. Xx t mn sin wf Natural frequency.
A 50-kg block moves between vertical guides as shown. What is the force necessary to pull the blocks to the right with an acceleration of 2 m s 2. F100N m50kg Therefore F - f ma In this case a0 Therefore F f f100N.
The 300-kg block is tied to a wall by a horizontal rope. A 5 kg block moves in a straight line on a horizontal frictionless surface under the influence of a force that varies with position as shown in the figure. F F 40gsin30 0 F 2626 N F block 2 3 does not slip relative to each other block 2.
A block of mass 2 kg slides along a horizontal tabletop. A block of mass 175 kg is pushed up against a wall by a force Pthat makes an angle of 500 angle with the horizontal as shown below. CHAPTER 8 ff PROBLEM 81 Determine whether the block shown is in equilibrium and find the magnitude and direction of the friction force when θ 25 and P 750 N.
If the coefficient of kinetic friction between the block and the table is 02 the frictional force exerted on the block is most nearly. W w w t p w n n n n n k m k 12000 12000 15 20 2 28 284 2 Nm Nm kg rads. The coe cient of static friction between the block and the wall is 0260.
For arrange The block is pulled 40 mm down ment b determine the period of of the vibration the maximum velocity of the block and the maximum acceleration block. A 50-kg block moves between vertical guides as shown. For each spring arrangement determine the period of vibration the maximum velocity and maximum acceleration of the block.
The scale of the figures vertical axis is set by F 600 N. A 50-kg block moves between vertical guides as shown. A 50 kg block moves in a straight line on a horizontal frictionless surface under the influence of a force that varies with position as shown in the figure.
Solved A 50 Kg Block Moves Between The Vertical Guides As Chegg Com
0 Comments